Géza Pattantyús-Ábrahám Doctoral School of Mechanical Engineering 

BME GPK, Department of Applied Mechanics

Supervisor: Dr. Dombóvári Zoltán

Modeling of machining processes with industrial robots

Introducing the research area

There is a growing interest in the industry to use robotic arms to manufacture metal parts. This can be a cost-friendly and versatile alternative to machine tools mostly in finishing operations. Robots face two main problems in terms of dynamics in these applications. Firstly, a robotic arm has a dynamically weak structure compared to dedicated machine tools and is prone to more significant nonlinear behavior [R1]. Secondly, machining processes like turning and milling are regenerative processes [R2] as the past states of the dynamic system play an important role in the long-term behavior of the system. This regenerative effect can cause large, so-called chatter vibrations, which almost always limit the stable process parameters. By addressing these issues and developing possible solutions, robotic machining can play an important role in the future of the machining industry.

Brief introduction of the research place

The research is carried out at the Department of Applied Mechanics of the Mechanical Engineering Faculty of BUTE and partly conducted in the MTA-BME Lendület Machine Tool Vibration Research Group. Experimental studies are partly conducted in cooperation with IDEKO, a member of the Basque Research and Technology Alliance, which is part of the prominent DANOBAT group. Parts of the research are also done in collaboration with the University of Bristol, Georgia Institute of Technology, and the Basque Center for Applied Mathematics.

History and context of the research

Robots were originally developed for simple pick-and-place [R3] operations, where extreme geometrical accuracy is usually not required. Later, industrial robots were used in some machining applications, especially for clay prototype manufacturing. By that time positioning accuracy was increased by an order due to better sensors and built-in proportional derivative control. This precision was sufficient for the aforementioned sculpture-like clay prototype manufacturing, where cutting forces are not significantly large. This role of robots was gradually taken over by 3D printing, but clay prototypes in some cases are still a cheap and durable solution to test design issues, e.g., sculpture-like car chassis prototypes for design purposes.

Nowadays, the machining of metal parts with industrial robots seems a promising option, mainly due to their versatility and relatively low cost compared to machine tools. Most common open kinematical chain robots are excellent for the manufacturing of sculpture-like surfaces and have a large workspace. The disadvantage of this versatile configuration is that it lacks rigidity – a key requirement in metal part machining – and they are prone to exhibit strong nonlinear behavior mainly attributable to the gear drives. Furthermore, the vibrational parameters of robots are pose-dependent and can vary on a large scale during machining, which makes them hard to characterize and numerically simulate experimentally.

The research goals, open questions

This research aims to prepare stock robots for the demanding task of metal cutting, which currently has many practical limitations. The correct dynamic characterization of the robotic arms is as important as having the mathematical tools to deal with the corresponding intricate problems. Today’s limitations in the research topic are both theoretical and experimental.

The introduced methods provide design options for robotic machining to ensure and improve stability as well as robustness during the operation. The main difficulties are the possible strong nonlinear stiffness characteristic arising mainly at the joints and drives, the instabilities originating in the machining (regenerative vibration) and delayed feedback controllers, and the time-varying vibrational parameters depending on the position of the end effector. These problems must be tackled step by step first, allowing a more unified approach, which can be used to develop machining applications for robots. As these phenomena can and do occur in various engineering problems, the presented mathematical tools can be of great value in other scientific research fields.

Methods

Additional acceleration feedback controller to improve dynamic performance.

The dynamic performance of robots needs to be upgraded for metal cutting. A simple approach would be to deploy an online control system using built-in velocity or acceleration feedback signals. Although this would be an easy solution as it does not require additional expensive equipment, it is still problematic since manufacturers usually do not provide access to their built-in controllers for safety reasons. Another approach to increase the dynamic reflected stiffness of the end effector is to use mounted passive or semi-active embedded systems. However, industrial robot users usually do not feel comfortable accepting these solutions. Using an additional acceleration controller besides the built-in PD controller of the robot can be a relatively cheap and simple solution to improve stability and robustness. To achieve stable manufacturing with the new feedback controller, careful parameter management and the synchronization of time delays of the controllers are required. Theoretically, stability can be improved and the possible harmful nonlinearities from the robotic configuration can be counteracted using nonlinear state feedback [P1].

 

Effect of quantization in digital controllers

The quantized nature of digital controllers can have a significant impact on the dynamical system because of the intricate periodic feedback signals. This is especially true for neutral and advanced systems [R4], where the state depends also on the derivative or the second derivative of past states. The time delay of these digital controllers can be modeled by sawtooth-like time-periodic functions. The time-periodic nature of the controllers together with the possible time periodicity of milling defines an important parameter called the principal period, which plays an essential role in the stability calculation of these systems.

 

Time-varying dynamical parameters of robots

The vibrational parameters of robots highly depend on the robot's position, which means that during the process these parameters can change on a large scale. The mathematical description results in nonautonomous (generally nonperiodic) dynamical systems with coefficients continuously varying in time. In this case, it may not be possible to achieve asymptotic stability over the entire time interval, e.g., the whole path traveled by the robot during machining. However, amplitude limits, i.e., finite time stability can be feasible. This requires the accurate prediction of the vibration amplitudes, which is not trivial for nonautonomous systems, and present state-of-the-art numerical methods lack the required precision. These requirements call for the development of an analytical approach that results in reliable solutions and provides a suitable basis for the verification of future numerical schemes.

Results

Hopf bifurcation of neutral delay differential equations

A two-degrees-of-freedom nonlinear robot model subject to acceleration feedback control was studied as shown in Fig. 1(a). We consider this as the simplest possible equivalent model that can reproduce the nonlinear behavior and also includes a nonlinear delayed feedback controller. The mathematical description leads to a neutral system.
Firstly, the linear stability of the mechanical system was studied (Fig. 1(b, c)). The nonlinear properties near the critical bifurcation point were calculated with the center manifold reduction derived for neutral equations [P2] and also verified with numerical continuation [R5]. The calculated nonlinear properties (Fig. 1(d, e)) show that the correct choice of nonlinear control parameters ensures stable limit cycles and improves robustness.

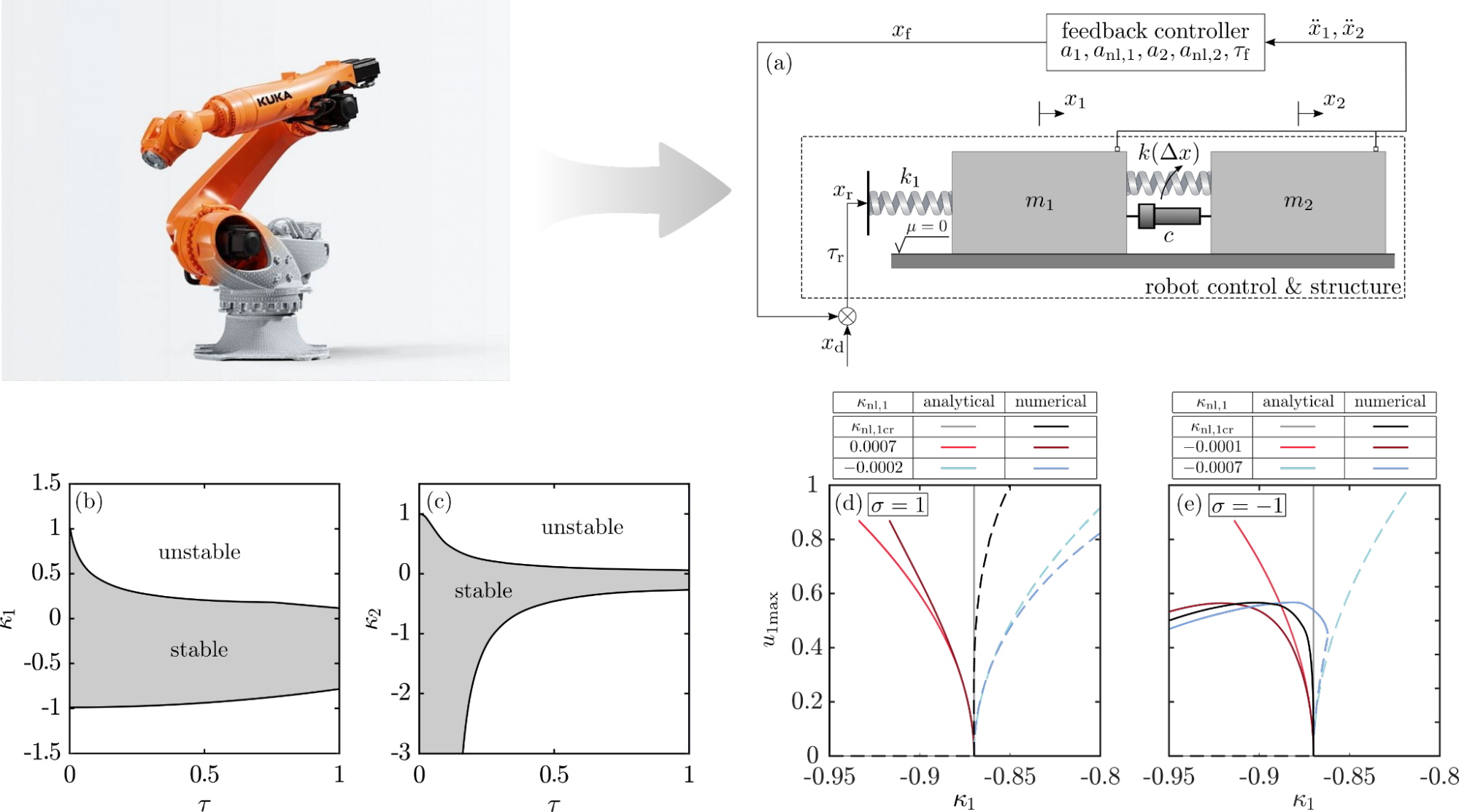
Figure 1: (i) two degrees-of-freedom robot model. (a,b) linear stability analysis. (c,d) analytical and numerical bifurcations for hardening (c) and softening (d) nonlinearities.

 

Stability of robotic machining with digital controllers

The semi-discretization method [R6] was generalized in a software package that can handle both quantized and continuous delays. For the approximation of the principal period, the Farey ratio was used. The numerical scheme was tested on two engineering applications.

First, the stability analysis of a hierarchical controller of an autonomous vehicle was completed. The theoretical calculations were in good agreement with the measurement results performed on a small-scale model [P3]. Secondly, the stability of a robotic arm in milling with built-in PD and additional acceleration controllers was analyzed [C2, P4]. The stability diagrams highlight that stability can be greatly improved using the proposed solution and the correct choice of feedback delay is essential to ensure good dynamic performance.

 

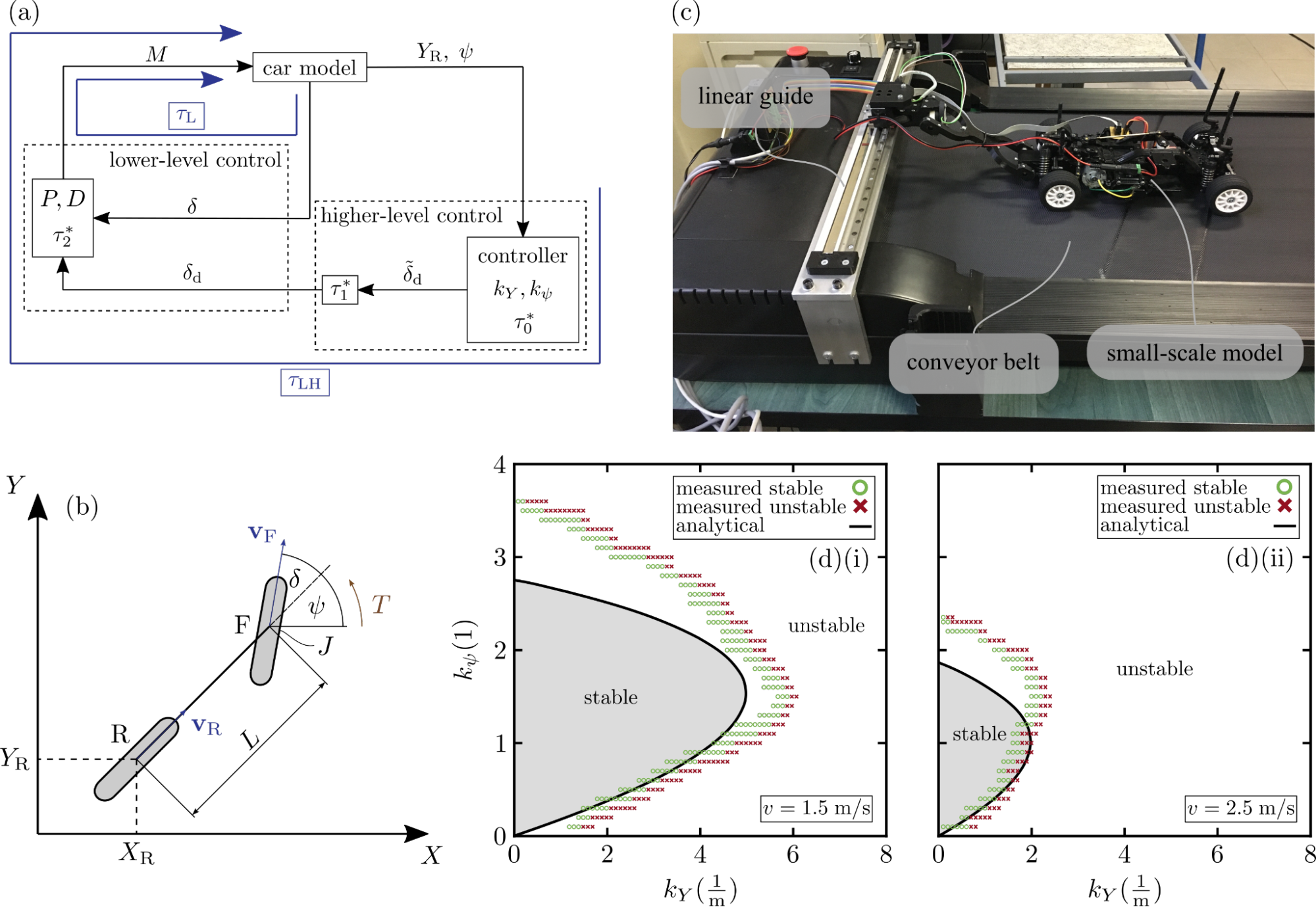
Figure 2: (a) hierarchical steering control. (b) bicycle model with rigid wheels. (c) measurement setup. (d) theoretical and experimental stability diagrams for different vehicle velocities.

 

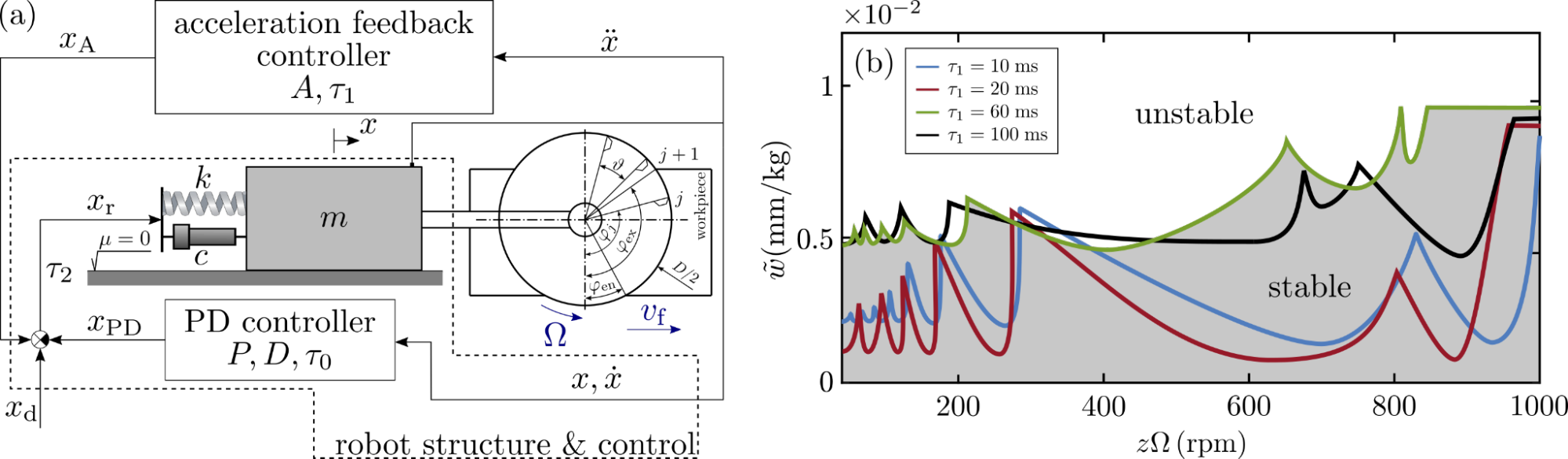
Figure 3: (a) robot structure in milling with additional acceleration feedback controller. (b) stability for different sampling time delays.

 

Analytical approximation of finite time stability of nonautonomous dynamical systems

To correctly approximate the critical vibration amplitudes of robots during the machining process, a framework for nonautonomous dynamical systems is required. For the homogeneous solution, the well-known WKB approximation [R7] can be used. For the particular solution, a novel concept based on iterative integration-by-parts was introduced [C1, P5]. The closed-form analytical formulas were evaluated on a practically relevant two-degrees-of-freedom dynamical system. In Fig. 4(ii)(a, b) it can be observed that classical numerical methods cannot correctly approximate finite time stability for large time spans, while Fig. 4(ii)(c, d) demonstrates the excellent precision of the analytical solution for small time spans as well.

 

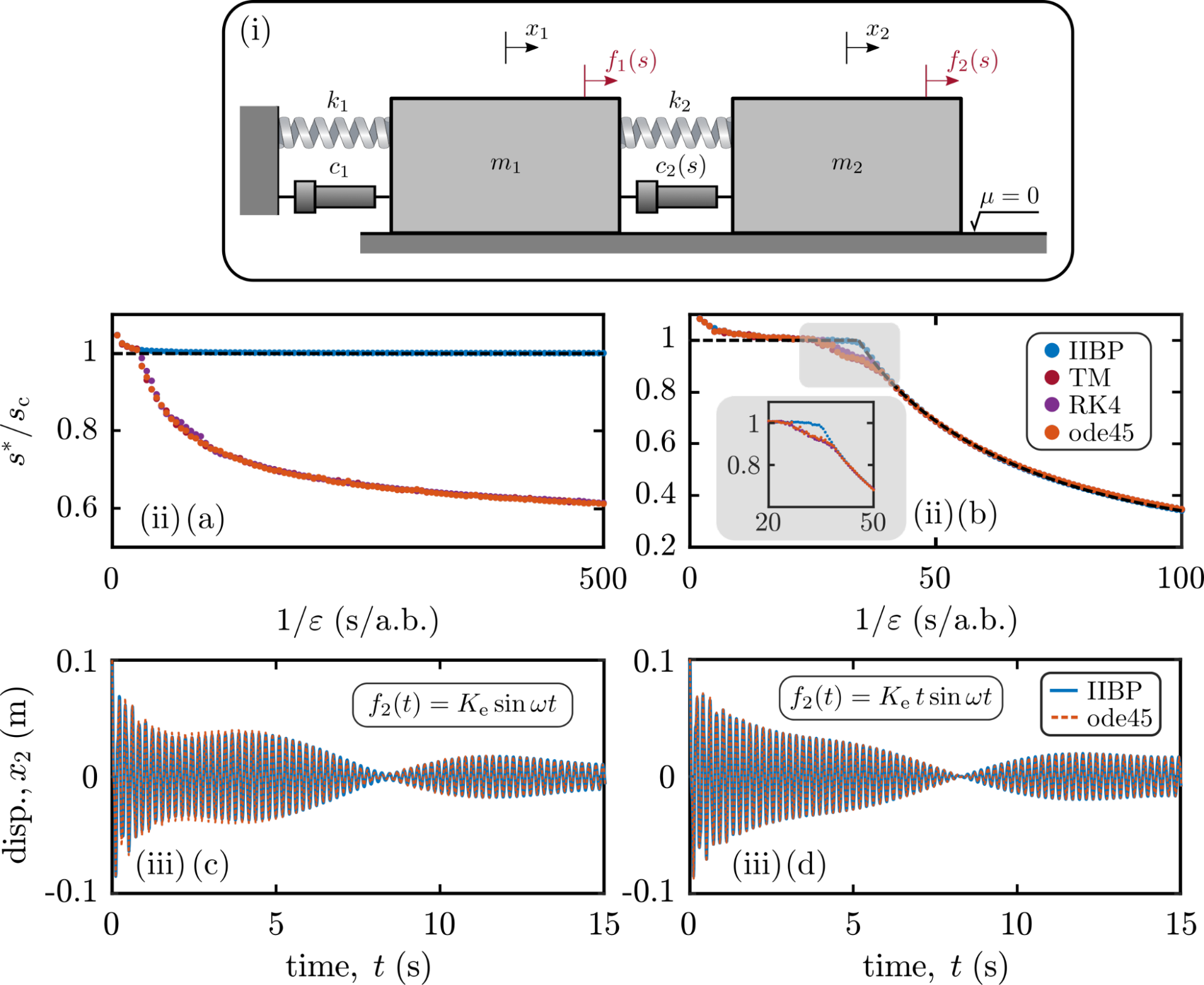
Figure 4: (i) two-degrees-of-freedom system with a time-varying damping parameter. (ii) novel analytical method compared to classical numerical schemes. (iii) time domain simulations.

Expected impact and further research

If the current difficulties in robotic machining can be overcome, then several machining tasks can be taken over from machine tools, significantly decreasing manufacturing cost and time. This leap from classical manufacturing can have a great impact on both serial production and prototyping.

The already achieved results of the research not only serve as important steps in accomplishing the metal cutting with robots, but they also provide general methods that can be used in other engineering problems. The most important aspect of future research is to successfully connect theoretically validated ideas with experimental data resulting in suitable industrial solutions.

Publications, references, links

List of corresponding own publications.

Journal papers.

[P1] Habib G., Bártfai A., Barrios A., Dombóvári Z. Bistability and delayed acceleration feedback control analytical study of collocated and non-collocated cases. Nonlinear Dynamics, 108, 2075–2096, 2022. (IF: 5.741)

[P2] Bartfai A., Dombovari Z., Hopf Bifurcation Calculation in Neutral Delay Differential Equations: Nonlinear Robotic Arms Subject to Delayed Acceleration Feedback Control. International Journal of Non-Linear Mechanics, 147, 2022. (IF: 2.985)

[P3] Bartfai A., Vörös I., Takacs D., Stability analysis of a digital hierarchical steering controller of autonomous vehicles with multiple time delays. Journal of Vibration and Control, OnlineFirst, 2023 (IF: 2.633)

[P4] Bartfai A., Barrios A., Dombovari Z., Stability Analysis of a One Degree-of-Freedom Robot Model with Sampled Digital Acceleration Feedback Controller in Turning and Milling. Journal of Computational and Nonlinear Dynamics, 18(8), 2023. (IF: 1.872)

[P5] Bartfai A., Ponce-Vanegas F., Kuske R., Hogan J., Dombovari Z., Framework for the analytical approximation of finite time stability of excited dynamical systems with time-varying parameters. SIAM Journal on Applied Mathematics, 2023. (under review, IF: 2.148)

[P6] Ponce-Vanegas F., Bartfai A., Dombovari Z., Semi-analytical solutions for slowly varying linear equations. (under submission)

 

Conference papers/Book chapters.

[C1] Bartfai A., Kuske, R., Dombovari, Z., On the finite-time stability of dynamic stability loss in a single degree of freedom system. In Proceedings of the ISMA2022 International Conference on Noise and Vibration Engineering, Leuven, 2565–2576, 2022.

[C2] Bartfai A., Barrios, A., Dombovari, Z., Stability Analysis of a One Degree-of-Freedom Robot Model with Sampled Digital Acceleration Feedback Controller in Turning. In Proceedings of the International Design Engineering Technical Conferences and Computers and Information in Engineering Conference (ASME IDETC-CIE2022), St. Louis, USA, 1–8, 2022.

[C3] Bartfai A., Dombovari Z., Demonstration of optimal tuning measurement of the tuneable clamping table with a polymer workpiece. 38th Danubia Adria Symposium on Advances in Experimental Mechanics, 2022, no. 3125.

[C4] Bartfai A., Dombovari Z., Bistability in nonlinear elastic robotic arms subject to delayed feedback control. 10th European Nonlinear Dynamics Conference (ENOC 2022), Lyon, France, 1113–1114, 2022.

[C5] Bartfai A., Barrios A., Dombovari Z., Robotic Machining Applications: Delayed Acceleration Feedback Control in Milling. Proceedings of the International Design Engineering Technical Conferences and Computers and Information in Engineering Conference (ASME IDETC-CIE2021), online, 2021, no. 73909.

[C6] Bártfai A., Dombóvári Z., A Case Study on the Stabilization of Advanced Delay Differential Equations with Digital Controllers. 15th IFAC Workshop on Time Delay Systems (TDS2021), online, pp. 234–235, 2021.

[C7] Bártfai A., Vörös I., Takács D., Időkésés és mintavételezés hatása egy autó sávtartó szabályozásában. XXIX. Nemzetközi Gépészeti Konferencia, Cluj-Napoca, Romania (OGÉT 2021), 204–207, 2021.

[C8] Iklodi Zs., Gabos Z., Bartfai A., Dombovari Z., Time Domain simulation of surface topography in broaching considering tools with general cutting-edge geometries and three DoF parametric dynamics. Manufacturing Engineering Society International Conference (MESIC 2023), Sevilla, Spain, pp. 3–12, 2023

[C9] Bartfai A., Dombovari Z., Demonstration of finite time stability in hardware-in-the-loop turning process with time-varying parameters. 39th Danubia Adria Symposium on Advances in Experimental Mechanics (DAS 39th), Siófok, Hungary, pp. 72–73, 2023

[C10] Bartfai A., Dombovari Z., Időben változó paraméterű esztergálás véges idejű stabilitás vizsgálatának numerikus szimuláció problémája. XIV. Magyar Mechanikai Konferencia, Miskolc, Hungary (MAMEK XIV.), 2023

 

Table of links.

Department of Applied Mechanics

MTA-BME Lendület Machine Tool Vibration Research Group

IDEKO

 

List of references.

[R1] de Luca A., Farina R., Lucibello P., On the control of robots with visco-elastic joints. In: Proceedings. 2005 IEEE International Conference on Robotics and Automation, 4297–4302, 2005

[R2] Munoa J., Beudaert X., Dombovari Z., Altintas Y., Budak E., Brecher C., Stepan G., Chatter suppression techniques in metal cutting. CIRP Annals 65(2), 785–808, 2016.

[R3] Hazarika S.M., Dixit U.S., Robotics: history, trends, and future directions, chap. 7. Materials Forming, Machining, and Tribology. Springer International Publishing, 213–239, 2018.

[R4] Insperger T., Stepan G., and Turi J., Delayed feedback of sampled higher derivatives. Philosophical Transactions of the Royal Society A 368(1911): 469–482, 2010.

[R5] Barton D.A. Krauskopf B., Wilson R.E., Collocation schemes for periodic solutions of neutral delay differential equations. Journal of Difference Equations and Applications, 12(11), 1087–1101, 2006.

[R6] Insperger T., Semi-Discretization for Time-Delay Systems. Springer-Verlag New York: Stability and Engineering Applications, 2011.

[R7] Baer S.M., Erneux T., Rinzel J., The slow passage through a Hopf bifurcation: Delay, memory effects, and resonance. SIAM Journal on Applied Mathematics, 49(1), 55–71, 1989.