Géza Pattantyús-Ábrahám Doctoral School of Mechanical Engineering Sciences 

BME Faculty of Mechanical Engineering, Department of Machine and Product Design

Supervisor: Dr. Kerényi György

Discrete Element Modelling on Maize

Introducing the research area

In parallel to the growing population of the Earth, our civilization is also continuously developing. Thus, more effective agricultural machineries with higher performance level are needed to produce even more food with higher level of quality (Figure 1).

In practice, the most common way of developing agricultural machineries is the method of 'trial and error' when the latest constructions are validated under in-situ conditions. This method is proved to be very expensive; moreover, thanks to the seasonal characteristic of agricultural crops its application is limited in time and space.

Nowadays, application of numerical simulations of the latest designs is a quite common way of reducing product development costs and extending the observation and analysis opportunities regarding the operational conditions. Contrary to this, a product development method that involves numerical simulations on the interactions between the agricultural crop and parts of the machine has not yet evolved in the field of agricultural machine development

Consequently, my PhD research focuses on the development of a numerical method that is capable of analyzing the interactions between the fibrous agricultural materials and the parts of the machine. A special fibrous material, harvest-ready maize, and a special process, harvesting with a combine harvester, were chosen for the study to justify the capability of the discrete element method.

 

Figure 1: Harvesting of wheat in the medieval age (left) and today (right) [L1, L2]

 

Brief introduction of the research place

My PhD research is conducted at the Department of Machine and Product Design (GT3) of the Faculty of Mechanical Engineering (GPK) at the Budapest University of Technology and Economics (BME), where education and research projects regarding agricultural machine development date back as early as the 1900s. As our industrial partners invest more and more into numerical methods, our research group focuses on the development of numerical methods to analyze agricultural machineries and process.

History and context of the research

Thanks to the rapid development of informational technologies, complex large-scale simulations which require high computational capacity are available today. In parallel to this, more and more numerical methods evolved and the Discrete Element Method (DEM) became the most wide-spread technique for modelling agricultural materials in practice [1].

DEM was developed to analyze the mechanical behaviour of particulate assemblies, thus, it was also adapted for modelling on granular agricultural materials, soil and grains, and processes that involve granular materials as soil cultivation [2], discharge of silos [3], drying of grains [4] and threshing [5].

Fewer studies can be found on the modelling of fibrous agricultural and forestry materials. Several possible DEM geometrical structures (chain of spheres, enhanced chain of spheres, hollow and solid model) were determined by Jünemann et al. [6]. A special model was developed to analyze the mechanical behaviour of wheat straws during transversal compression and three-point bending experiments by Leblicq et al. [7, 8]. The interaction between grass fibers and a disc-mower, the energy requirement of mowing and the cutting process were analyzed by Kemper et al. [9]. Cotzee and Lombard analyzed the process of destemming and the selection technique of grapes [10]. The effect of impact loads on woody branches was analyzed by Olmedo et al. [11].

The literature review clearly demonstrates that the previously developed measurement methods and DEM models focus on a special mechanical behaviour of the material under scrutiny, however, usually a complex phenomenon take place during the real agricultural process.

The research goals, open questions

The main goal of my research is to develop a discrete element model on the harvest-ready maize for qualitative and quantitative analysis on the complex process of maize harvesting by a combine harvester that can be integrated into the product development of agricultural machineries involved in maize harvesting.

During the research the following hypotheses will be justified regarding the maize plant and the DEM model:

●     a measurement method can be established that is capable to provide the necessary amount of quantitative and qualitative data about the characteristics of maize;

●     strong correlation can be found between the physical and morphological traits of maize;

●     strong correlation can be found between the physical properties of different morphological elements of maize and their mechanical behaviour;

●     the discrete element method is capable of forming the physical and mechanical model of maize;

●     a calibration method can be established that is suitable for the calibration and verification of the physical and mechanical parameters of the DEM model;

●     model parameters that have a significant effect on its mechanical behaviour can be determined;

●     the verified DEM model of maize is capable of analyzing the complex process of maize harvesting by a combine harvester;

●     the results provided by the model can be advantageously exploited during the product development.

Methods

A complex measurement method was formed to justify the hypotheses regarding the physical, morphological and mechanical traits of maize that involves the following experiments and observations [K1, K2]:

●     analysis of the soil condition of the experimental plot;

●     analysis of the meteorological parameters (mean air temperature, rainfall, solar radiation) of the experimental plot;

●     measurement of the geometrical traits of maize;

●     measurement of the physical properties (moisture content, mass) of maize;

●     cantilever bending experiment on different morphological elements of maize stalk;

●     three-point bending experiment on different morphological elements of maize stalk;

●     four-point bending experiment on different morphological elements of maize stalk;

●     sectional transversal compression experiment on different morphological elements of maize stalk;

●     local transversal compression experiment on different morphological elements of maize stalk;

●     dynamic cutting experiment on different morphological elements of virgin and pre-compressed maize stalk;

●     uniaxial tensile experiment on different morphological elements of maize stalk;

●     ear-detachment experiment on the harvest-ready maize;

●     ear-collision experiment on the crop of harvest-ready maize;

●     observations regarding maize harvesting.

 

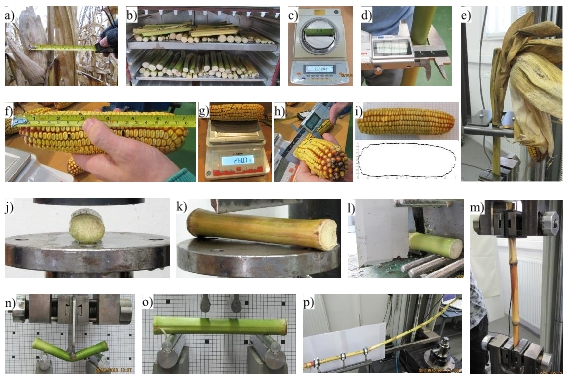
Figure 2: Measurement method to determine the main physical and mechanical characteristics of maize: a) position of maize ears; b) moisture content of maize stalk; c) mass of internodal sections of maize stalk; d) cross-sectional area of internodal sections of maize stalk; e) ear-detachment experiment; f) length of maize ears; g) mass of maize ears; h) diameter of maize ears; i) shape of maize ears; j) sectional transversal compression experiment; k) local transversal compression experiment; l) dynamic cutting experiment; m) uniaxial tension experiment; n) three-point bending experiment; o) four-point bending experiment; p) cantilever bending experiment [K1, K2]

 

Based on the measurement results, the discrete element model of maize was formed that involved the idealized root system, stalk and one maize ear. To determine the model parameters of different morphological elements an extensive parametric study was carried out on the model of the 4th internodal section of the stalk (Figure 3) by using the virtual models of sectional transversal compression, three-point bending and dynamic cutting experiments [K3, K4, K5].

 

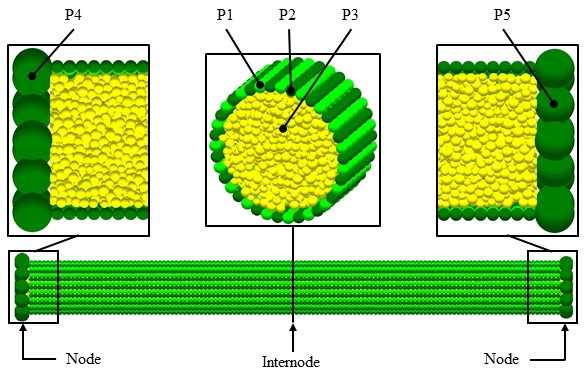
Figure 3: DEM model of the 4th internodal section which consists of the skin (P1 and P2), the core (P3) and the node (P4 and P5)

 

To predict the mechanical behaviour of the plant and complex interactions between the parts of the plant and parts of the machine with high accuracy, a calibration and verification method was established based on experiments; see in Figure 4 [K6, K7, K8].

 

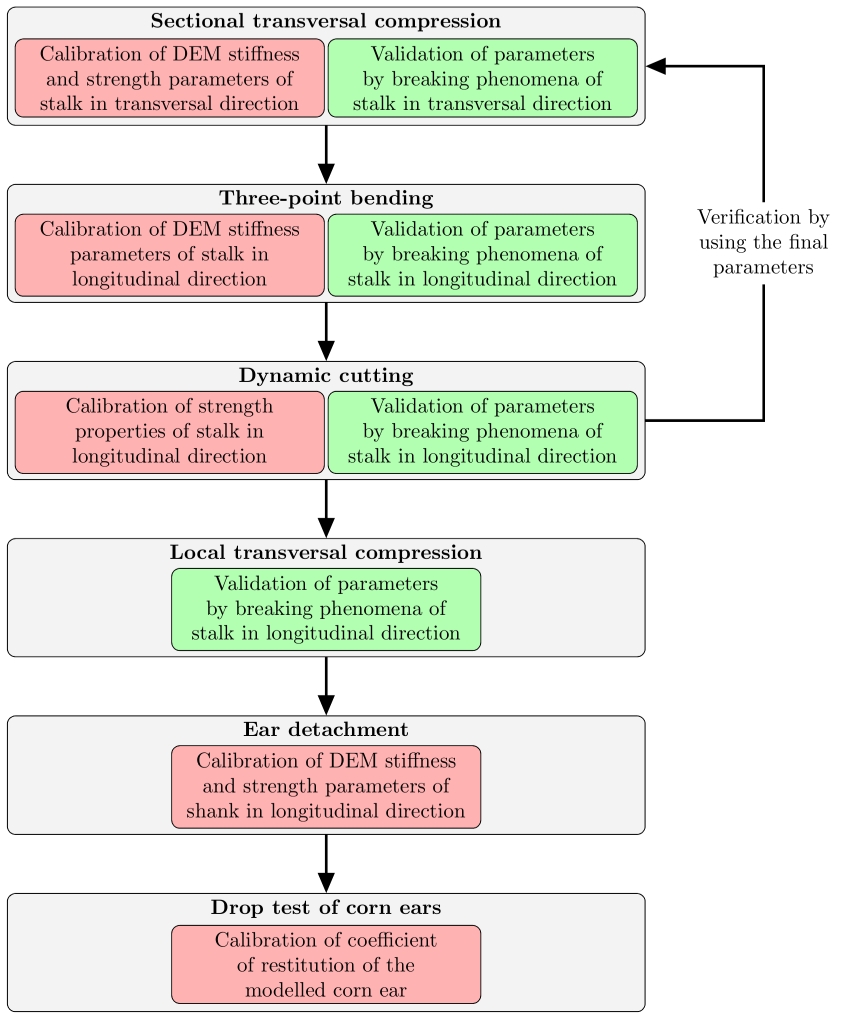
Figure 4: Calibration and verification method for the determination of DEM model parameters [K6]

 

Finally, a technological simulation on maize harvesting will be carried out by using the virtual model of a common maize header (Figure 5) and the calibrated and verified DEM model of maize [K9, K10].

 

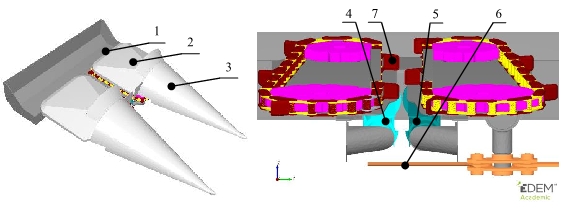
Figure 5: Virtual model of a common maize header:

1: gathering box; 2: hood; 3: row-divider; 4-5: left and right stalk roller; 6: chopping unit; 7: gathering chains [K9]

Results

During the measurements and observations several physical, morphological traits, and mechanical behaviour were analyzed and defined that was not measured earlier. The main findings are as follows [K1, K2].

●     the equivalent diameter of the internodal parts of maize stalk shows a bi-linear decrease from the bottom to the top of the plant, where the inflection point is the 6th internode, the position of the maize ear;

●     the wet mass of the first five internodes gives ~85% of the total wet mass of the stalk, thus, these parts provide the main part of the biomass;

●     the axisymmetric shape of an ideal maize ear was determined by using image processing methods;

●     the required compressive, bending and cutting work were determined for each internodal section, moreover, the characteristics of these parameters show a decreasing tendency from the bottom to the top of the maize stalk, so that the first five internodes provide the 80% of the total required compressive, bending or cutting work of a common stalk;

●     typical breakage of internodal parts were determined through transversal compression, bending and cutting;

●     the required ear-detachment force was determined;

●     the coefficient of restitution of maize ears was determined on different surfaces.

To determine the model parameters that have a significant effect on the mechanical behaviour of the model in case of different loading cases an extensive parametric study was carried out on the 4th internodal section. The main findings are summarized in Figure 6. [K3, K4, K5, K6]

 

 

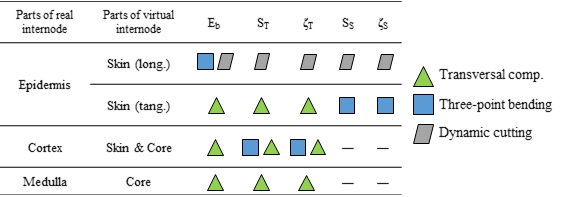
Figure 6: Relationship between the model parameters and loading cases:

E_b: bond stiffness; S_T: bond tensile strength; ζ_T: coefficient of variation, tensile; S_S: bond shear strength; ζ_S: coefficient of variation, shear;

 

To numerically predict the mechanical behaviour of the real internodal section during different loading cases, a parameter set was determined by using the calibration and verification method and the results of the parametric study.

Figure 7 presents the experimental and numerical results of sectional transversal compression. The force response of the DEM model is in good agreement with the experimental results. Moreover, the breakage of the virtual sample is similar to the observations during the experiments. [K4, K5, K6]

 

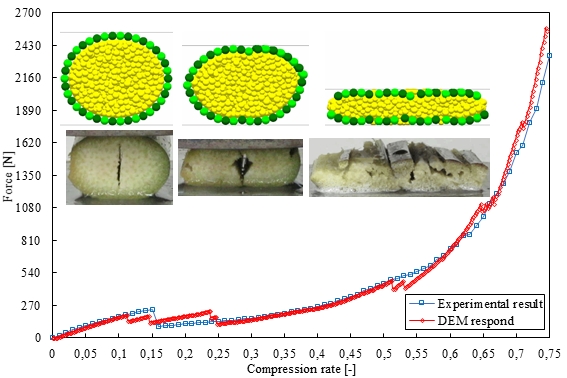
Figure 7: Experimental and numerical results of sectional transversal compression [K6]

 

Figure 8 presents the experimental and numerical results of three-point bending experiment. The force response of the DEM model is in a good agreement with the experimental results but for the last section. This can be attributed to the elastic mechanical behaviour of the applied bonded model. Moreover, the breakage of the virtual sample looks similar to the observations during the experiments. [K4, K5, K6]

 

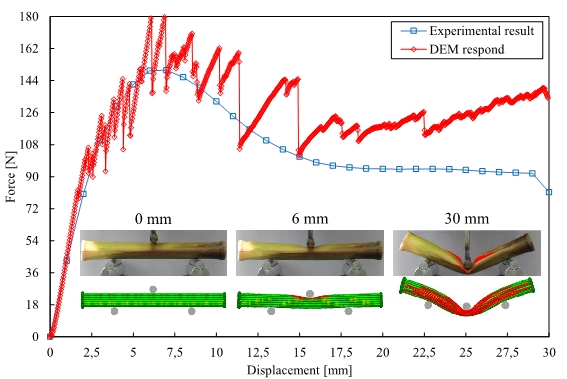
Figure 8: Experimental and numerical results of three-point bending [K6]

 

Figure 9 presents the experimental and numerical results of the dynamic cutting experiment. During the virtual cutting process, the same stages of cut can be found: initial compression, cutting and sliding as in the case of the real process. Moreover, the virtual cutting surface is really similar to the experimentally observed ones. [K4, K5, K6]

 

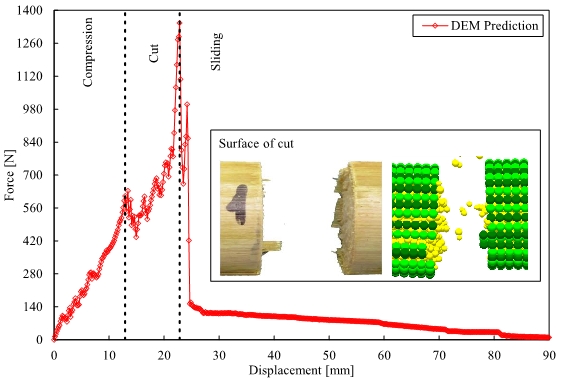
Figure 9: Experimental and numerical results of dynamic cutting [K6]

 

Expected impact and further research

Using the established measurement method, several physical, morphological and mechanical characteristics of the maize plant were determined that were not measured or observed before. The experimental results can be advantageously exploited for virtual modelling, breeding and crop production.

The presented DEM model of maize is capable of analyzing the interactions between parts of the plant and part of the machine; moreover, it can predict the complex mechanical behaviour of the real plant during different loading cases.

During the further research, the focus will be put on the entire plant model. By using the results from the parametric study and the calibration and validation method, the rest of the plant will be formed. Finally, a large-scale technological simulation will be carried out on maize harvesting and the results will be analyzed.

Publications, references, links

List of corresponding own publications:

 

[K1] Kovács, Á., Jóri, I.J., Gaál, K., Piros, A., Kerényi, G., 2015. Development of measurement methods for a numerical simulation of corn plants. Mechanical Engineering Letters, Szent István University, 13, 88-96.

 

[K2] Kovács, Á., Jóri I.J., Kerényi G., Gaál K., 2015. Mérési módszerek kifejlesztése kukorica növény numerikus szimulációjához. Mezőgazdasági Technika, LVI. évf. (12) 2-5.

 

[K3] Kovács, Á., Kerényi, G., 2016. Comparative analysis of different geometrical structures of discrete element method (DEM) for fibrous agricultural materials. 4th CIGR International Conference of Agricultural Engineering (CIGR-AgEng2016). Aarhus, Denmark.

 

[K4] Kovács, Á., Kotrocz, K., Kerényi, G., 2015. The adaptability of discrete element method (DEM) in agricultural machine design. Hungarian Agricultural Engineering, 27, 14-19. https://doi.org/10.17676/HAE.2015.27.14

 

[K5] Kovács, Á., Kerényi, G., 2016. Stochastic variation in discrete element method (DEM) for agricultural simulations. Hungarian Agricultural Engineering, 30, 31-38. https://doi.org/10.17676/HAE.2016.30.31

 

[K6] Kovács, Á., Kerényi, G., 2018. A new method to calibrate discrete element models of fibrous agricultural materials. European Agricultural Engineering Conference (AgEng 2018). Wageningen, the Netherlands, July 8-12, 2018.

 

[K7] Kovács, Á., Rádics, P. J., Kerényi, G., 2017. A discrete element model for agricultural decision support. 31st European Conference on Modelling and Simulation (ECMS 2017). Budapest, Hungary, May 23-May 26, 2017.

 

[K8] Kovács, Á., Kerényi, G., 2017. Modeling of corn ears by discrete element method (DEM). 31st European Conference on Modelling and Simulation (ECMS 2017). Budapest, Hungary, May 23-May 26, 2017.

 

[K9] Kovács, Á., Zwierczyk, P.T., 2018. Coupled DEM-FEM simulation on maize harvesting. 32nd European Conference on Modelling and Simulation (ECMS 2018). Wilhelmshaven, Germany, May 22-May 25, 2018.

 

[K10] Kovács, Á., Zwierczyk, P.T., Kerényi, G., 2017. Kapcsolt véges és diszkrét elemes szimulációk (FEA-DEM) alkalmazása a mezőgazdasági géptervezésben. XXV. Nemzetközi Gépészeti Találkozó, ISSN 2068-1267. Kolozsvár, Romania, 2017.

 

Table of links:

 

[L1] Wheat harvesting in the mediaeval age

 

[L2] Wheat harvesting today

 

List of references:

 

[1] Cundall, P.A., Strack, O.D.L., 1979. A discrete numerical model for granular assemblies. Géotechnique 29, 47-65. https://doi.org/10.1680/geot.1979.29.1.47

 

[2] Tamás, K., Jóri, I.J., Mouazen, A.M., 2013. Modelling soil-sweep interaction with discrete element method. Soil and Tillage Research 134, 223-231. https://doi.org/10.1016/j.still.2013.09.001

 

[3] Coetzee, C.J., Els, D.N.J., 2009. Calibration of discrete element parameters and the modelling of silo discharge and bucket filling. Computers and Electronics in Agriculture 65, 198-212. https://doi.org/10.1016/j.compag.2008.10.002

 

[4] Keppler, I., Kocsis, L., Oldal, I., Farkas, I., Csatar, A., 2012. Grain velocity distribution in a mixed flow dryer. Advanced Powder Technology 23, 824-832. https://doi.org/10.1016/j.apt.2011.11.003

 

[5] Yu, Y., Fu, H., Yu, J., 2015. DEM-based simulation of the corn threshing process. Advanced Powder Technology 26, 1400-1409. https://doi.org/10.1016/j.apt.2015.07.015

 

[6] Jünemann, D., Kemper, S., Frerichs, L., 2013. Simulation of stalks in agricultural processes - Applications of the Discrete Element Method. Landtechnik 68, 164-167.

 

[7] Leblicq, T., Smeets, B., Ramon, H., Saeys, W., 2016. A discrete element approach for modelling the compression of crop stems. Computers and Electronics in Agriculture 123, 80-88. https://doi.org/10.1016/j.compag.2016.02.018

 

[8] Leblicq, T., Smeets, B., Vanmaercke, S., Ramon, H., Saeys, W., 2016. A discrete element approach for modelling bendable crop stems. Computers and Electronics in Agriculture 124, 141-149. https://doi.org/10.1016/j.compag.2016.03.022

 

[9] Kemper, S., Thorsten, L., Frerichs, L., 2014. The overlaid cut in a disc mower - results from field tests and simulation. Landtechnik 69, 171-175.

 

[10] Coetzee, C.J., Lombard, S.G., 2013. The destemming of grapes: Experiments and discrete element modelling. Biosystems Engineering 114, 232-248. https://doi.org/10.1016/j.biosystemseng.2012.12.014

 

[11] Olmedo, I., Bourrier, F., Bertrand, D., Berger, F., Limam, A., 2016. Discrete element model of the dynamic response of fresh wood stems to impact. Engineering Structures 120, 13-22. https://doi.org/10.1016/j.engstruct.2016.03.025