 |
BMe Research Grant |
|
Doctoral School of Physics
Department of Physics/Institute of Physics
Supervisor: Dr. Simon Ferenc
Theoretical and Experimental Investigation of Spin Dynamical Phenomena in Carbon Based Nanostructures
Introducing the research area
It appears that the physical limitations of the conventional semiconductor based computer technology is already realized by companies, like Intel [1] and are counting on spintronics [2] as a possible future alternative. The use of spintronics based devices is impossible without understanding the basic physical processes, such as spin relaxation and spin decoherence. In addition, the investigation of new, mainly nanostructured materials potentially applicable to spintronics is intensively researched. During my PhD research, I prepare and investigate new candidate materials for use in spintronics base transistors. I examine the fundamental nature of spin relaxation properties of these new materials like graphene and carbon nanotubes.
Brief description of the research place
The research takes place at the ESR Laboratory of the Department of Physics at the BUTE, equipped with devices for the preparation of new nanostructured materials and modify their chemical and physical properties (Ar filled box, vacuum system). The measurement equipment and methods include electron spin resonance spectrometer (ESR), NMR, microwave resistance measurements, Raman spectroscopy, optical fluorescence, optically detected magnetic resonance. Additionally, the laboratory develops own instruments as well. The outstanding liaisons with external research groups allows doing experiments that require experimental capabilities not available in our lab, e.g. Prof. L. Forró: EPF Lausanne, Lausanne, Switzerland; Prof. T. Pichler: Universität Wien, Vienna, Austria; Prof. N. M. Nemes: Universidad Complutense de Madrid, Madrid, Spain; Prof. J. Fortágh: Universität Tübingen, Tübingen, Germany; Dr. Ferenc Murányi: IT’IS, Zürich, Switzerland; Prof. A. Hirsch: Universität Erlangen-Nürnberg, Erlangen, Germany; Prof. J. Fabian: Universität Regensburg, Regensburg, Germany; Prof. S. Reich: Freie Universität Berlin, Berlin, Germany; Prof. M. Riccò: Università Degli Studi di Parma, Parma, Italy.
There are close connections with some local groups as well: Prof. Jenő Kürti (Eötvös University); Prof. Balázs Dóra (BUTE, Department of Theoretical Physics).
History and context of the research
The currently used silicon based technology in computer science is approaching its limit of applicability, therefore, it is imperative to look for architectures with new principles and new materials. One of the possible candidates is spintronics, where the information is coded in electron spins. Data transmission realized this way would theoretically result smaller and more efficient devices. The principles of a transistor based on spintronics, the SFET is presented in Fig. 1.
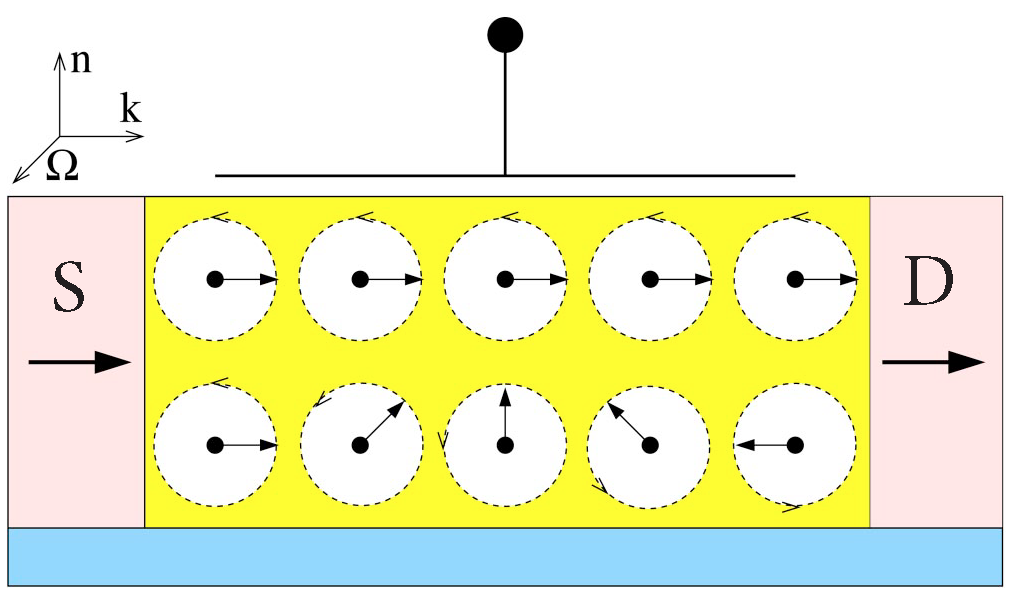
Figure 1: Theoretic realization of the SFET. The S Source and the D detector (Drain) is made of ferromagnetic material. The spins of the injected spin polarized electrons precess with
angular frequency in the transfer medium. This frequency can be manipulated through the spin-orbit coupling with the upper gate. If the spin arriving to D has parallel orientation with D (upper row) then large, if anti-parallel (bottom row) a small current will flow in the transistor [1]. The spin orientation is highly influenced by the transfer medium as well, because the injected spins lose their orientation due to spin relaxation caused by the spin-orbit coupling
The importance of the material between the source and the detector can be recognized from the figure above. To avoid the loss of information the spin relaxation time – the amount of time until the spin orientation is detectable – has to be as long as possible. The modern low dimensional carbon nanostructures, such as graphene, carbon nanotubes, fullerenes and their chemically modified species have promising properties from this point of view [3, 4, 5, 6, 7].
The research objectives, open questions
One of the goals of the research is to find a material, where the so-called spin diffusion length is the longest possible, to preserve the spin information as long as it is possible in the device. The diffusion length is proportional to square root of the product of the electron mobility and the spin relaxation time, thus one has to look for a material with high mobility and long spin relaxation time. Graphene and other carbon allotropes are promising due to their weak spin-orbit coupling and high mobility, yet they entail loads of challenges. According to theoretical calculations regarding graphene [4], the spin relaxation time reaches the 10–100 ns range required for applications, however, this range cannot be verified experimentally so far. [3, 8]. Most probably, the primary reason for the discrepancy is the relaxation on the contacts and the spin relaxation induced by the substrate [9]. To exclude the effects of contacts and substrate, a contactless method like ESR spectroscopy can be applied. Unfortunately, ESR investigation of graphene is hindered by the low sensitivity of detectors (even on the biggest samples) and the low density of states in graphene at the Fermi energy. Both of them can be bypassed by chemical modification (doping, functionalization), where the Fermi energy is shifted and the number of investigated spins can be multiplied. A further opportunity may be the investigation of intercalated graphite and doped nanotube systems which possess the same electron structure as graphene; these can be easily examined with bulk spectroscopic methods [10, 11, 12, S1, S2]. Single walled carbon nanotubes as rolled up graphene stripes enable the investigation of curvature effects, too. Interestingly, DFT calculations predict that certain types of doped graphene become superconductors, although neither carbon, nor alkali- and alkali earth metals feature this property [13]. The refinement of theoretical models and their application to the emerging systems is also part of the research, just like the investigation of anisotropic spin relaxation.
Methods
Sample preparation:
The multi-layer graphene samples used during the research were prepared by the group of Prof. Andreas Hirsch within a cooperation, whereas the remaining materials were bought from commercial companies. The alkali doping was carried out with the use of the Ar filled box and vacuum systems in our lab with any of the 3 possible methods: (i) vapor phase, (ii) molten immersion, (iii) liquid ammonia solution [14, 15, 16]. In case of vapor phase intercalation the quartz tube is narrowed at the middle to form two chambers: one contains the carbon material and the dopant is placed in the other. On heating the ends to different temperatures, the emerging temperature gradient causes material flow. This method can be applied for doping all investigated materials, however, only with dopants with high enough vapor pressure and they may not diffuse into quartz (eligible dopants: Na, K, Rb, Cs, Yb). In case of molten immersion, the sample is placed directly into the molten metal. An important condition here is that the sample has to be crystalline. The reaction is carried out in a copper (or steel) tube, therefore, intercalation with lithium and calcium is also possible. The third, liquid ammonia solution method is based on the dissolubility of alkali metals in liquid ammonia. The setup applied is presented in Fig. 2. Mixing the solution for a while forces the metals to penetrate into the material to be doped. The technique is well suited for powders but works great with graphite as well [S3]. Unfortunately, saturation
stoichiometry cannot be assured in every cases.
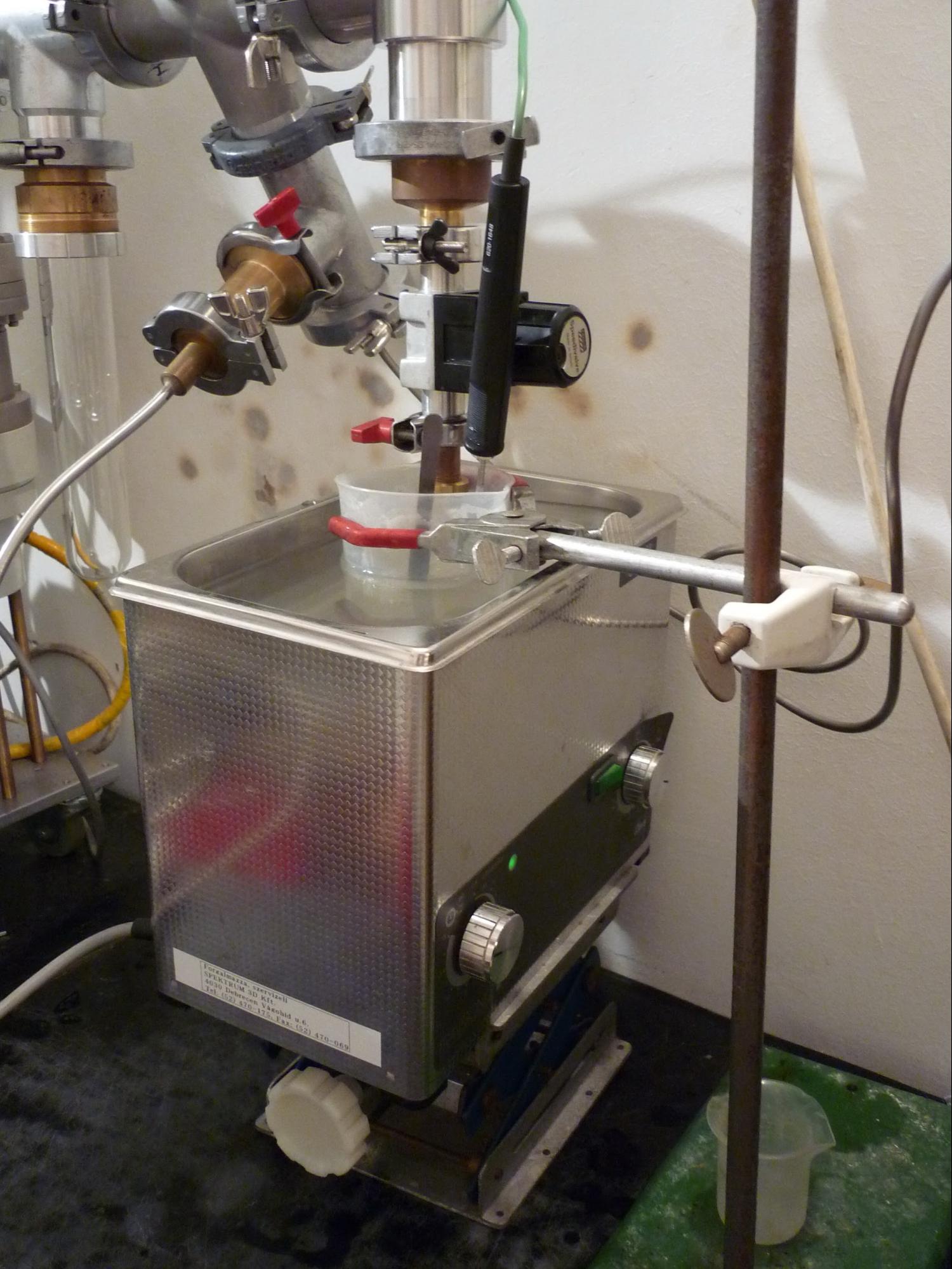
Figure 2: The setup used during the liquid ammonia solution method. During the preparation, the sample is immersed in liquid ammonia, in an ethanol bath and in an ultrasonicator, while its temperature is maintained at -50°C
Experimental techniques:
In the field of spintronical research, electron spin resonance spectroscopy has outstanding importance. An advantage of the technique is that it allows contactless examination and through utilizing the Zeeman-effect it gives a straightforward way of determining the spin relaxation time through the dynamic susceptibility, which can be derived from the waveform. For small field (< 1 T) measurements, a commercial Bruker Elexsys E500 is used and for high field values we use a homemade spectrometer [17]. The low temperature and various high field measurements are carried out at the EPFL within a cooperation. Verification of the theoretical models might require knowledge on the resistance values of the created materials. A microwave conductivity measurement setup being developed ongoing in our lab is used to provide this information [18, S4]. Furthermore, within a cooperation with the Universität Wien, the samples are investigated with Raman-spectroscopy, which provides important information about the quality of doping through the evaluation of vibrational spectrum. This technique provides information about the optical phonons close to center of the Brillouin zone. In the case of electron-phonon systems strongly coupled in the spectrum, a specific waveform (Breit-Wigner-Fano) can be observed in the spectrum as a result. Through theoretical models, electron-phonon coupling constant, an important value in testing superconductivity, can be determined from this [19].
Results
In the first part of my work I characterized the few layer graphene (FLG) starting material with the methods mentioned above. I investigated the effect of mechanical post-processing as well in this case, which was ultrasound sonication (US), shear mixing (SM) and magnetic stirrer (ST) [S5]. In Fig. 3 the Raman results show that the received samples are not perfect monolayers, but contain few layer components as well. The G mode is in perfect agreement with the monolayer graphene, the 2D mode can be divided into 2 components, and only one fits with the results of the monolayer [20].
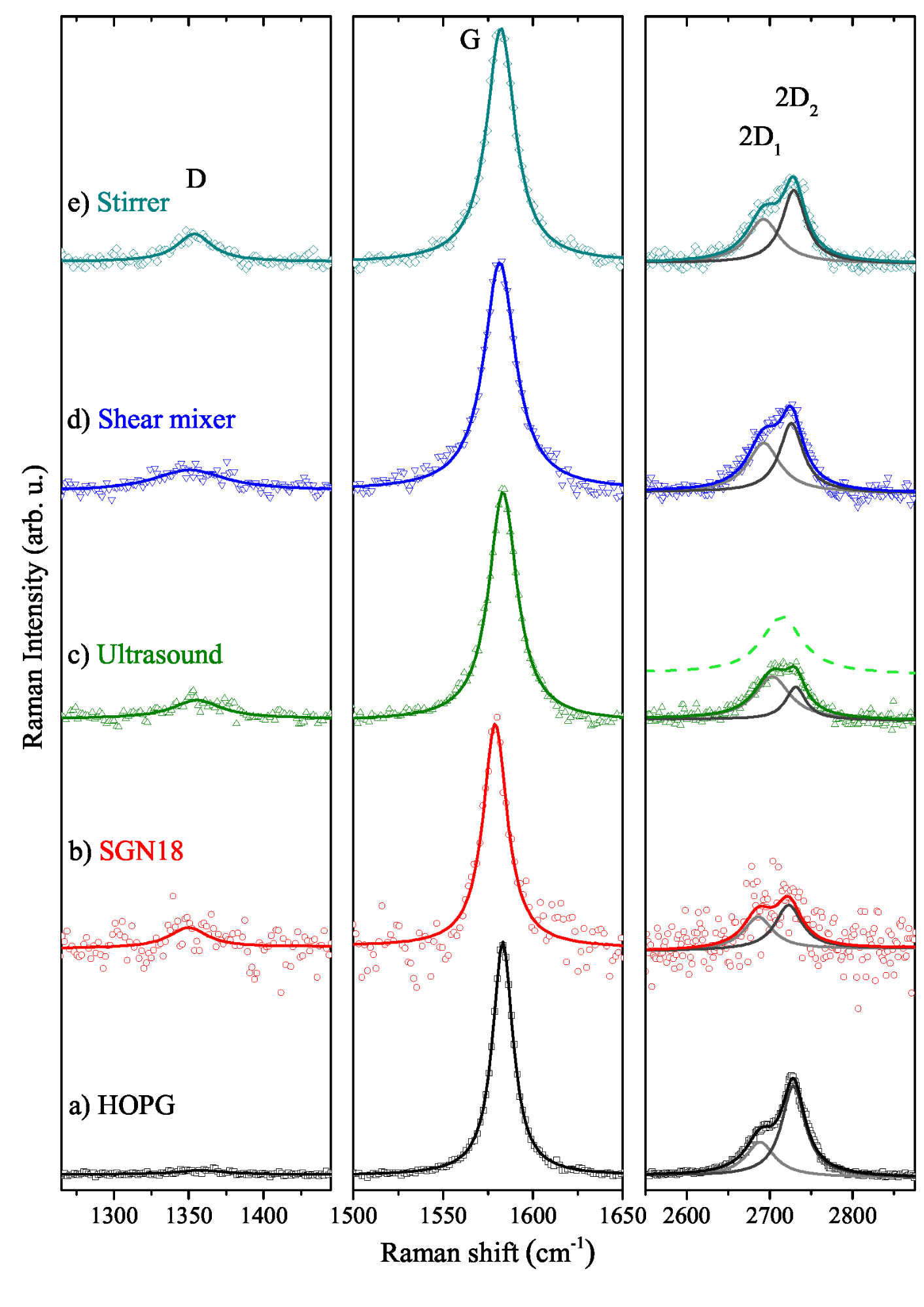
Figure 3: D, G and 2D Raman modes of chemically exfoliated graphene samples with different mechanical post processing. a) bulk HOPG, b) graphite powder, c) US, d) SM, e) ST graphene. The gray lines mark the division of the 2D peak. In case of the ultrasound sonicated sample, the 2D peak can be fitted with one curve only (dashed curve)
The results of the ESR measurements are shown in Fig. 4. As a result we got that the samples are slightly p-doped, which is not surprising knowing the reaction scheme. The spin relaxation time is about 1–3 ns.
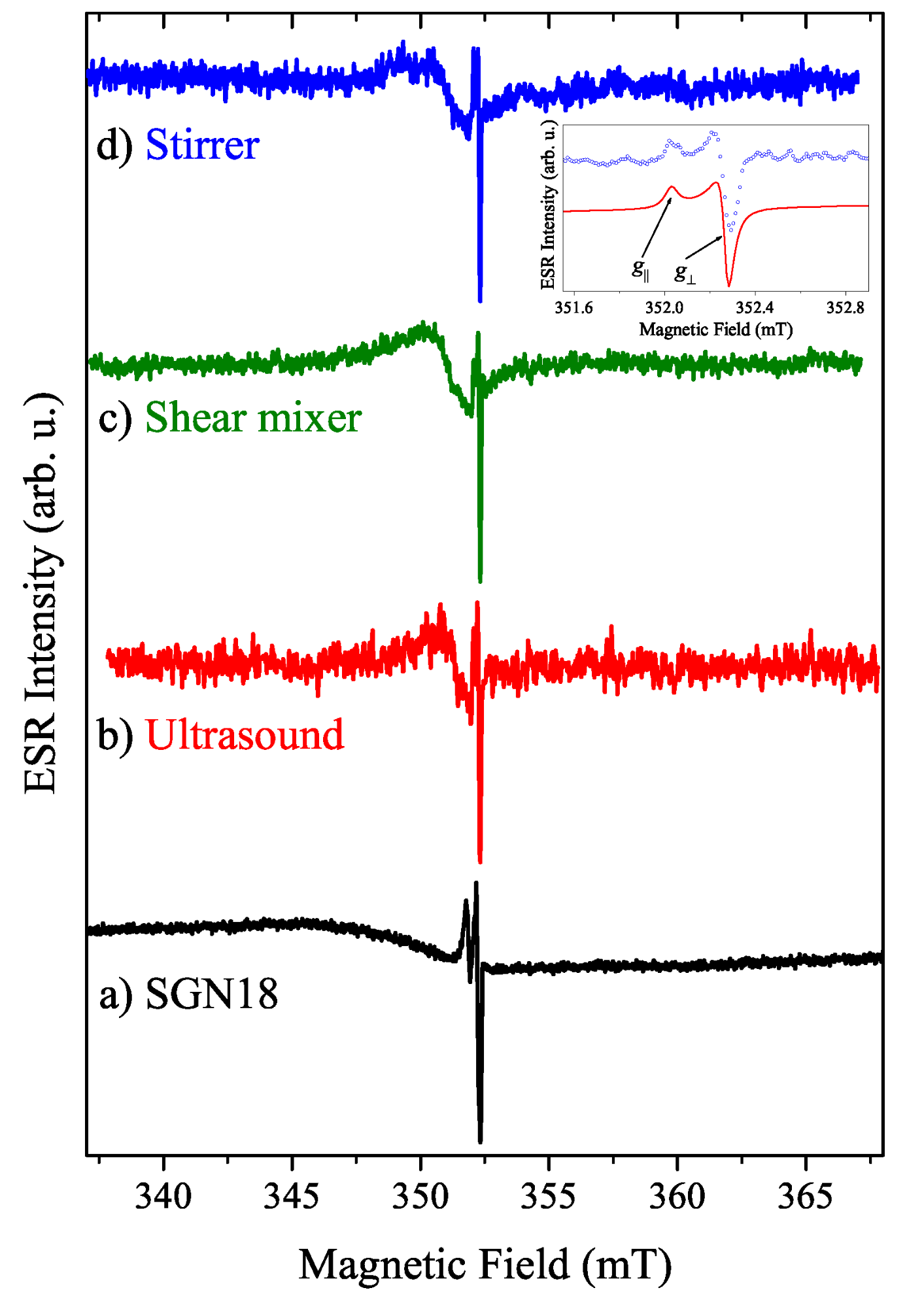
Figure 4.: ESR spectra of the investigated materials. In the graphite powder a broad (12.2 mT) feature is present at g=2.0148, as expected. In the US graphene a 1.1 mT broad line is found at g=2.0059 with the shape of a derivative Lorentzian. In the SM at g=2.0082 with the width of 1.4 mT the same behavior was observed. The ST sample present a feature with a uniaxial anisotropy and with the width of 1.2 mT and a position of g=2.0094. Inset shows the signal for the uniaxial signal with a fit for the narrow line in case of the ultrasounded sample.
We observed with microwave resistivity measurements that the resistivity is quite different, especially at low temperatures. The most likely explanation is that it is caused by the different grain sizes. We concluded that the quality of the received sample was good, it contained one and few layer graphene flakes. The best result is provided by ultrasound treatment. As a further step, we investigated what happened with the Raman response upon continuous doping with potassium. The results revealed that new, mobile charge carriers appeared in the material [S6, S7]. We also found that graphene flakes of 3–5 layers were the most frequent. The measurements were repeated with samples placed on SiO2 and hBN, which showed similar behavior, presented in Fig. 5 [S8, S9].
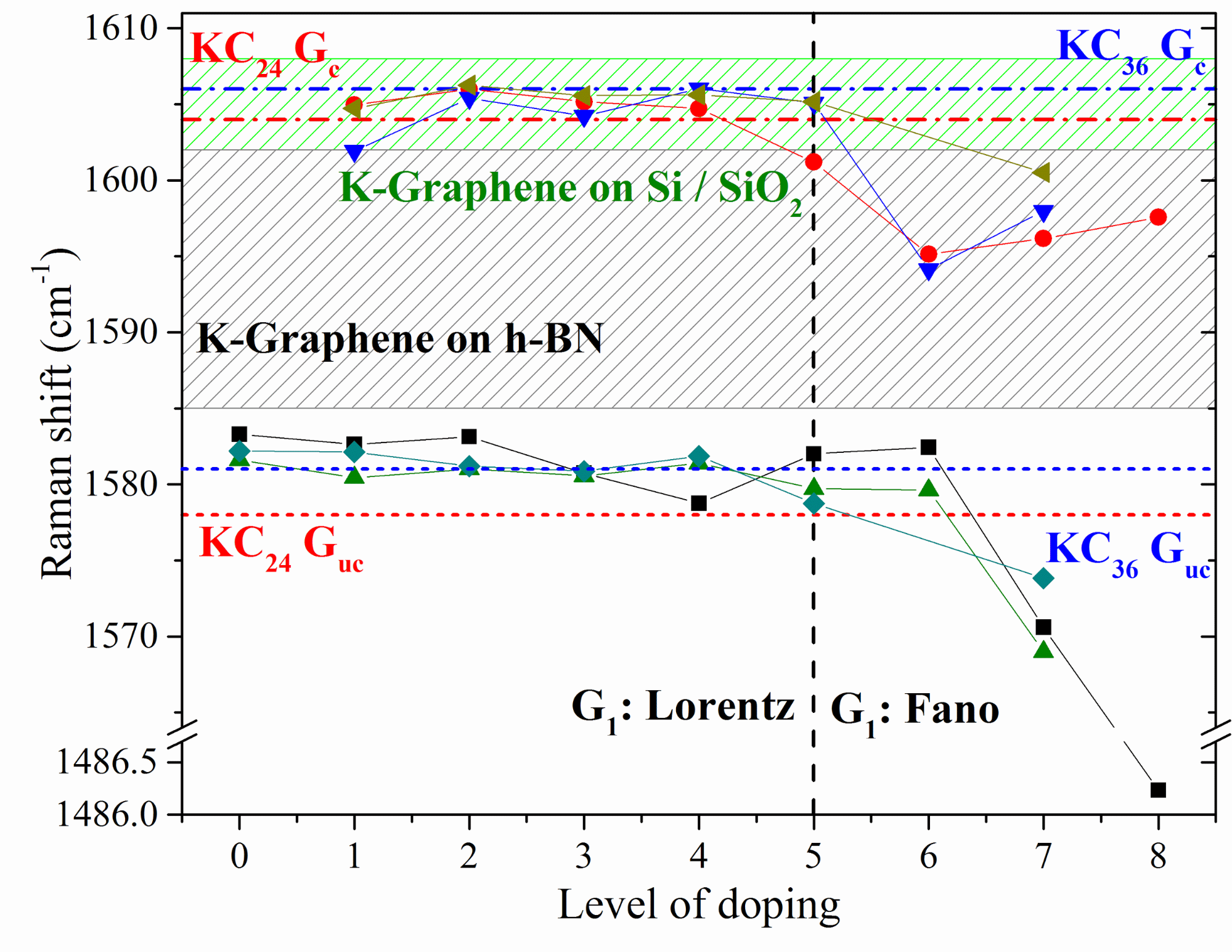
Figure 5: Behavior of the G mode of the graphene samples upon potassium doping. On the left side of the vertical line the G1 (G mode belonging to the charged layers) peak has Lorentzian shape, while on the right it has BWF-shape. Shading marks the results for the samples placed on different substrates
In case of ESR measurements, the investigation of continuous doping is not possible, but Li and Na doping works well. Upon lithium doping, we observed two peaks associated with conducting electrons. The narrower peak, according to literature is associated with the few layer, while the broader is associated with the monolayer phase. The spin relaxation time varies between 5–10 ns for the two lines, which is a big improvement over the originating material. Sodium doping is interesting, because graphite cannot be intercalated with it. Accordingly, the Na doped FLG only presents one ESR line which belongs to the monolayer component and is a strong proof that there are graphene monolayers in the sample. The spin relaxation time for this system is about 12 ns. Spin relaxation in anisotropic systems, such as KC8 [S1], LiC6 [S10], CaC6 and graphite is also part of our investigations. The goal is to generalize existing theoretical models for these systems, which are currently not available. The Li4C60 superionic conductor was also a subject of our research [S11], and whether a similar phenomena can be observed for lithium doped carbon nanotubes [S12].
Expected impact and further research
The upcoming task is publishing our results regarding the FLG system. The results give information to chemist colleagues about the preparation techniques, so the recipe can be refined to produce better quality samples. From the physics point of view, it is important to understand the materials before applying them in spintronics and this requires the longest spin relaxation time possible. Once completed, the first experiments with device preparation can be launched, which can be considered a huge step towards industrial application. The refinement of theoretical models and the explanation of new phenomena constitute an essential part of physics, so they are unavoidable. The superionic materials are primarily intended for use in rechargeable batteries, where lithium-graphite cells are currently being used. Switching to a similar material featuring superior properties, therefore, can be quick and smooth.
Publications, references, links
Publications:
[S1] B. G. Márkus, L. Szolnoki, D. Iván, B. Dóra, P. Szirmai, B. Náfrádi, L. Forró, F. Simon: Anisotropic Elliott-Yafet Theory and Application to KC8 Potassium Intercalated Graphite, submitted to publication: Physica Status Solidi B (2016)
[S2] P. Szirmai, B. G. Márkus, B. Dóra, G. Fábián, J. Koltai, V. Zólyomi, J. Kürti, L. Forró, T. Pichler, F. Simon: Density of states in potassium doped carbon nanotubes; a model system of biased graphene, in preparation to publish in ACS Nano
[S3] B. G. Márkus, F. Simon: Alkali Intercalation of Highly Ordered Pyrolytic Graphite in Ammonia Solution, 18th Conference of Czech and Slovak Physicists, conference proceeding, ISBN 978-80-244-4726-1 (2014)
[S4] B. Gyüre, B. G. Márkus, B. Bernáth, F. Murányi, F. Simon: A time domain based method for the accurate measurement of Q-factor and resonance frequency of microwave resonators, Rev. Sci. Instrum. 86, 094702 (2015)
[S5] B. G. Márkus, F. Simon, J. C. Chacón-Torres, S. Reich, P. Szirmai, B. Náfrádi, L. Forró, T. Pichler, P. Vecera, F. Hauke, A. Hirsch: Transport, magnetic and vibrational properties of chemically exfoliated few-layer graphene, Physica Status Solidi B 252 (11), 2438–2443 (2015)
[S6] P. Szirmai, J. C. Chacón-Torres, B. G. Márkus, P. Vecera, J. M. Englert, U. Mundloch, A. Hirsch, B. Náfrádi, L. Forró, C. Kramberger, T. Pichler, F. Simon: Raman spectroscopy for the probe of the layer number in exfoliated graphene, in preparation to publish in ACS Nano
[S7] B. G. Márkus, P. Szirmai, J. C. Chacón-Torres, P. Vecera, F. Hauke, A. Hirsch, J. M. Englert, T. Pichler, L. Forró, S. Reich, F. Simon: Synthesis and Electronic Properties of Li-doped Chemically Exfoliated Graphene, IWEPNM 2015, conference poster (7–14 March 2015)
[S8] J. C. Chacón-Torres, B. Hatting, S. Heeg, C. Berger, C. Woods, B. G. Márkus, F. Simon, A. Vijayaraghavan, S. Reich: Optical properties of highly doped graphene, Graphene Week 2015, conference poster (22–26 June 2015)
[S9] J. Chacón, B. Hatting, S. Heeg, B. G. Márkus, F. Simon, C. Berger, A. Vijayaraghavan, S. Reich: Vibrational response of graphene in the highly-doped regime, IWEPNM 2016, conference poster (13–20 February 2016)
[S10] B. G. Márkus, D. Iván, L. Szolnoki, B. Dóra, P. Szirmai, B. Náfrádi, L. Forró, F. Simon: Anisotropic Spin Relaxation in Graphite Intercalated Compounds, IWEPNM 2016, conference poster (13–20 February 2016)
[S11] D. Quintavalle, B. G. Márkus, A. Jánossy, F. Simon, G. Klupp, M. A. Győri, K. Kamarás, G. Magnani, D. Pontiroli, M. Riccò: Electronic and ionic conductivities in superionic Li4C60, Physical Review B 93, 205103 (2016)
[S12] B. G. Márkus, P. Szirmai, T. Pichler, F. Simon: Lithium doped Single-walled carbon nanotubes, 18th Conference of Czech and Slovak Physicists, conference poster (16–19 September 2014)
Links:
Home page of the Budapest University of Technology and Economics
Home page of the Department of Physics
References:
[1] K. Bourzac: Intel: Chips Will Have to Sacrifice Speed Gains for Energy Savings, MIT Technology Review (2016)
[2] I. Žutić, J. Fabian, S. Das Sarma: Spintronics: Fundamentals and applications, Reviews of Modern Physics 76 (2), 323–410 (2004)
[3] N. Tombros, Cs. Jozsa, M. Popinciuc, H. T. Jonkman, B. J. van Wees: Electronic spin transport and spin precession in single graphene layers at room temperature, Nature 448, 571–574 (2007)
[4] B. Dóra, F. Murányi, F. Simon: Electron spin dynamics and electron spin resonance in graphene, Europhysics Letters 72 (1), 17002 (2010)
[5] W. Han, R. K. Kawakami, M. Gmitra, J. Fabian: Graphene spintronics, Nature Nanotechnology 9, 794–807 (2014)
[6] K. Tsukagoshi, B. W. Alphenaar, H. Ago: Coherent transport of electron spin in a ferromagnetically contacted carbon nanotube, Nature 401, 572–574 (1999)
[7] R. Lina, F. Wanga, M. Wohlgenannta, C. Heb, X. Zhaib, Y. Suzuki: Organic spin-valves based on fullerene C60, Synthetic Metals 161 (7–8), 553–557 (2011)
[8] W. Han, R. K. Kawakami: Spin Relaxation in Single-Layer and Bilayer Graphene, Physical Review Letters 107, 047207 (2011)
[9] C. Ertler, S. Konschuh, M. Gmitra, J. Fabian: Electron spin relaxation in graphene: The role of the substrate, Physical Review B 80, 041405(R) (2009)
[10] A. Grüneis, C. Attaccalite, A. Rubio, D. V. Vyalikh, S. L. Molodtsov, J. Fink, R. Follath, W. Eberhardt, B. Büchner, T. Pichler: Electronic structure and electron-phonon coupling of doped graphene layers in KC8, Physical Review B 79, 205106 (2009)
[11] A. Grüneis, C. Attaccalite, A. Rubio, D. V. Vyalikh, S. L. Molodtsov, J. Fink, R. Follath, W. Eberhardt, B. Büchner, T. Pichler: Angle-resolved photoemission study of the graphite intercalation compound KC8: A key to graphene, Physical Review B 80, 075431 (2009)
[12] G. Fábián, B. Dóra, Á. Antal, L. Szolnoki, L. Korecz, A. Rockenbauer, N. M. Nemes, L. Forró, F. Simon: Testing the Elliott-Yafet spin-relaxation mechanism in KC8: A model system of biased graphene, Physical Review B 85, 235405 (2012)
[13] G. Profeta, M. Calandra, F. Mauri: Phonon-mediated superconductivity in graphene by lithium deposition, Nature Physics 8, 131–134 (2012)
[14] M. S. Dresselhaus, G. Dresselhaus: Intercalation compounds of graphite, Advances in Physics 51 (1), 1–186 (2002)
[15] D. Guerard, A. Herold: Intercalation of lithium into graphite and other carbons, Carbon 13 (4), 337–345 (1975)
[16] C. A. Kraus: Solutions of metals in non-metallic solvents; i. general properties of solutions of metals in liquid ammonia, J. Am. Chem. Soc. 29 (11), 1557–1571 (1907)
[17] K. L. Nagy, D. Quintavalle, T. Fehér, A. Jánossy: Multipurpose High-Frequency ESR Spectrometer for Condensed Matter Research, Applied Magnetic Resonance 40 (1), 47–63 (2011)
[18] B. Nebendahl, D.-N. Peligrad, M. Požek, A. Dulčić, M. Mehring: An ac method for the precise measurement of Q-factor and resonance frequency of a microwave cavity, Rev. Sci. Instrum. 72, 1876 (2001)
[20] J. C. Chacón-Torres, L. Wirtz, T. Pichler: Manifestation of Charged and Strained Graphene Layers in the Raman Response of Graphite Intercalation Compounds, ACS Nano 7 (10), 9249–9259 (2013)
[21] A. C. Ferrari, J. C. Meyer, V. Scardaci, C. Casiraghi, M. Lazzeri, F. Mauri, S. Piscanec, D. Jiang, K. S. Novoselov, S. Roth, A. K. Geim: Raman Spectrum of Graphene and Graphene Layers, Physical Review Letters 97, 187401 (2006)